Walking the Moon with my own Photos - How Small Objects Can You Recognize on the Moon?

Introduction | What Does the Naked Eye See on the Moon? | What Do I See with my Telescopes on the Moon? | What Do I See with my Telescopes on the Moon? | What Does a Digital Camera at a Telescope See on the Moon? | What Does a Digital Camera Alone See on the Moon? | References

On these pages I "walk the moon" on the basis of my own photos. In other words, I try to name the objects on my lunar photos to get to know the moon better. Maybe these pages will help others to get to know the moon better as well...

On this page, I ask how small objects can be on the moon that you you still can recognize them. See also page Moon Walks which provides an overview of the "moon walks" and some information about the moon.

 

Introduction

When you take photos of the moon, of course, the question arises how small objects you can see or recognize on photos. Since I do not take enough time with visual observations and do not inform myself properly beforehand, the question remains, what can be seen on the photos. A quick empirical answer is that, in my photos, you can see minimum objects with a diameter of 10 to 20 km. The image resolution seems to depend less on the magnification (also through the camera optics), but rather on the telescope itself, i. e. its aperture. Since the aperture determines the resolution of telescopes, my first impression is no surprise.

In the following, I will extend the question to the eye, visual observation with the telescope, the Atik Infinity camera at the telescope, and a digital camera at the telescope using the 1:50 or projection method.

 

What Does the Naked Eye See on the Moon?

Estimation: Diameter of the moon = 3476 km; field of view of approx. 30' (0.5°) => 3476 / 30' = 115.86 km/' > 1.93 km/" => about 120 km/' = 2 km/" => 1 km = 0.5" (apogee: 29'10" > 119.2 km/'; perigee: 33,5' > 103.76 km/'

Thus, with a lunar diameter of 3,476 km and a field of view of approx. 30' (0.5°) = 1800", an arc second would result in a distance of approx. 2 km (1.93 km).

The theoretical resolution of the eye is given as 20", which corresponds to 38.6 km on the moon. In practice, however, only 60" = 1' are achieved, which corresponds to 115.87 km. For astronomical observations, even lower values for the resolution of the eye are assumed, namely 2' or 3'. This provides us with the following "selection" of targets for the naked eye:

Now everybody can check for him- or herself what can be recognized on the moon with the naked eye! In the end, I think that I need a meaningful value for the resolution of the eye at the moon...

 

What Do I See with my Telescopes on the Moon?

Now I want calculate the theoretical resolution of my telescopes! Usually, the resolution on a telescope is calculated according to the formulae of Rayleigh and Dawes, which are based on different criteria for the resolution. In practice, telescope manufacturers provide only the value of the resolution according to the Dawson formula. It is solely based on the aperture of the telescope and is calculated as follows:

Dawes criterion: Telescope resolution (") α = 116 / Aperture (= diameter of the objective lens or main mirror in mm)

We already know that an arc second corresponds to a distance of about 2 km (1.93 km). Depending on the aperture, my telescopes (at the tme that I created this page...) have a theoretical resolution between 1.61" (2.8" tube) and 0.77" (6" tube). This would correspond to moon structures between 1.5 and 3.5 km, which are considerably smaller than I can perceive in practice. Now the question is, whether my eye is able to resolve that. To answer this, I need to know which magnification is used, because depending on which resolution I assume for the eye, the moon structures have to be between 20" and 180" in size (or even larger) so that I can recognize them.

Let us assume a magnification of 100 x, a field of view for the moon of about 30' (0.5°) = 1800" and a diameter of 3476 km for the moon. Thus, we get 50° = 3000', spread over 3476 km. This results in

Thus, with a resolution of 180" (3') I already come close to the theoretical resolution of my smallest telescope (1.61" according to Dawson). However, this is only achieved at the beneficial magnification, which is typically 1.5 times the aperture in mm. For a 4" telescope, the beneficial magnification is about 150 x; here we get 75° = 4500', spread over 3476 km. This results in:

At this magnification, 180" (3') is also sufficient to get close to the theoretical resolution of my 4" telescope (1.15" according to Dawes).

Resolutions for the Moon

However, the moon is not a double star to which the resolution values according to Dawes and Rayleigh refer, but a bright, flat object on which certain structures are located. Accordingly, in their book Moonhopper, Spix & Gasparini provide two resolution formulas from practice for the moon, which resemble the Dawes formula, but contain smaller numerical values and thus lead to lower resolutions/higher magnifications:

According to the authors, these are, however, theoretical resolution values that have to be doubled due to practical conditions (air turbulence, quality deficits of the telescope) most of the nights.

In their book, Spix & Gasparini determine the resolutions and the corresponding distances on the moon for a number of telescope apertures. I tried to reproduce this for my telescopes, but found some small deviations in the numerical values. The following table presents the resolution values (practical values for the moon) that I calculated and the corresponding theoretical distances on the moon for my telescopes:

Telescope Focal Length
of Telescope		 Aperture Beneficial Magnification
Resolving Power
Moon Km
Dawes Craters Grooves Dawes Craters Grooves
PS 72/432
432
72
108
1.74"
1.08"
0.64"
3.36
2.09
1.23
Heritage 100P
400
100
150
1.15"
0.78"
0.46"
2.22
1.51
0.89
Skymax-102
1300
102
153
1.15"
0.76"
0.45"
2.22
1.48
0.87
Skymax-127
1500
127
190.5
0.91"
0.61"
0.36"
1.76
1.19
0.70
Explorer 150PDS
750
150
225
0.77"
0.52"
0.31"
1.49
1.00
0.59

Thus, 4" telescopes should be able to show objects having a size between 900 m (grooves) and 1.5 km (craters) on the moon.

Magnifications

So the question arises under whether and how these values can be achieved in practice. According to what I understood so far with respect to magnifications, this should be the case for exactly the beneficial magnification (the resolutions of the telescope and the eye match), which has to be adjusted accordingly for grooves and craters (i.e. to be higher). Spix & Gasparini's formulae result in the following magnification formulae for a resolution of 1' (60", concentrated observing), which they assume:

Note: I tried to understand how the values in the authors' book are generated and found that they do not double the factor 39 or 23 in their calculations (as they do when presenting resolution values).

Based on an eye resolution of 1', Spix & Gasparini calculate the beneficial magnifications for the moon for a number of apertures. The following table does the same for my telescopes, as well as for different combinations of my eyepieces and telescopes, and presents magnifications and distances on the moon (I also present the doubled "practical" distances):

Telescope Aperture Resolving
Power
(Dawes) 		Beneficial
Magnific.
(Dawes) for 1'/2'/3'*		 FL of
Telescope
mm 		FL of
Eyepiece
mm 		Magni-
fication 		M** Enlarged
Diameter '
km on the Moon***
1' 2' 3' 4'
PS 72/432 72 1.61" 36/72/108
432
32
12.50
E
405.00
8.58
17.17
25.75
34.33
24
16.67
E
540.00
6.44
12.87
19.31
25.75
7
61.71
E
1851.43
1.88
3.75
5.63
7.51
11.60
37.24
bM
1117.24
3.11
6.22
3.90
110.77
C
3323.08
1.05
2.09
2.30
187.83
G
5634.78
0.62
1.23
Heritage 100P 100 1.15" 50/100/150
400
32
12.50
E
375.00
9.27
18.54
27.81
37.08
24
16.67
E
500.00
6.95
13.90
20. 86
27.81
7
57.14
E
1714.29
2.03
4.06
6.08
8.11
7.73
51.73
bM
1551.72
2.24
4.48
2.60
153.85
C
4615.38
0.75
1.51
1.53
260.87
G
7826.09
0.44
0.89
Skymax-102 102 1.15" 51/102/153
1300
32
40.63
E
1218.75
2.85
5.70
8.56
11.41
24
54.17
E
1625.00
2.14
4.28
6.42
8.56
7
185.71
E
5571.43
0.62
1.25
1.87
2.50
24.64
52.76
bM
1582.76
2.20
4.39
8.28
156.92
C
4707.69
0.74
1.48
4.89
266.09
G
7982.61
0.44
0.87
Skymax-127 127 0.91" 63.5/127/190.5
1500
32
46.88
E
1406.25
2.47
4.94
7.42
9.89
24
62.50
E
1875.00
1.85
3.71
5.56
7.42
7
214.29
E
6428.57
0.54
1.08
1.62
2.16
22.83
65.69
bM
1970.69
1.76
3.54
7.68
195.38
C
5861.54
0.59
1.19
4.53
331.30
G
9939.13
0.35
0.7
Explorer 150PDS 150 0.77" 75/150/225
750
32
23.44
E
703.13
4.94
9.89
14.83
19.77
24
31.25
E
937.50
3.71
7.42
11.12
14.83
16
46.88
E
1406.25
2.47
4.94
7.42
9.89
7
107.14
E
3214.29
1.08
2.16
3.24
4.33
9.67
77.79
bM
2327.59
1.49
2.99
3.25
230.77
C
6923.08
0.50
1.0
1.92
391.30
G
11739.13
0.30
0.59

*) Based on Spix & Gasparini's book Moonhopper, where they use an eye resolution of 1' (beneficial magnification = aperture in mm / 2) for all telescope types, I use a resolution of 1' for the calculations. These values are not identical with the "bM" values where I used the exact Dawes factor of 116 (in this column, I use 120 for "simplicity). I also provide "rounded" beneficial magnifications for the typically used resolutions of 2' and 3'.
**) E = Magnification calculated from the focal lengths of the telescope and the eyepiece, bM = beneficial magnification (1'), C = craters (1'), G = grooves (1')
***) The italic values in column "2'" correspond to doubling the constants in the resolution formulae given by Spix & Gasparini in their book Moonhopper; these are the values that are to be compared with the values calculated above!
Magnifications in italic: These values are given in Spix & Gasparini's book Moonhopper and serve as a cross check for my calculations

Comparison of moon distances: Luckily, the distances here (the italic values) match the values calculated above!

Discussion

Distances: Regarding the "practical" distances on the moon, the above table does not provide any new information. The distances are very small (1 - 2 km for 4" telescopes), and I find it hard to believe them at all. I will therefore in the future try to check, which distances I can actually recognize with the individual telescopes, using exact moon maps for guiding my observations. This will definitely take a while...

Magnifications: The beneficial magnifications for craters are about in the order of magnitude of what I previously knew as "maximum usable magnification," which is, however, based on a resolution of 3'. I certainly tried these magnifications already, and so, using a new approach, I just landed where I was before... For grooves, however, the beneficial magnifications are significantly higher. As a test, I already operated the Skymax-102 with a magnification of more than 300 x at the Sinus Iridum (Golden Handle), which was quite OK under the given conditions. In plain language this means, and as Spix & Gasparini write, you have to try, try, try... According to the authors, magnifications of more than 350 x can hardly be used in Central Europe.

My eyepieces: When observing the moon, I often use my the 32 mm and 24 mm eyepieces together with the Skymax tubes, the former one for photos, because it has a T-connector, the latter one for visual observations, where it displays the moon as a whole. According to the table above, depending on the resolution of the eye (or "concentrated" versus "comfortable" observation), moon distances of less than 10 km should be visible. So far, I have not checked whether I can actually see such distances with these eyepieces - another task for the future. I can almost photograph them, but I am more likely to recognize structures of a size between 10 and 20 km away (see below).

Notes on Spix & Gasparini's Book Moonhopper

First, I had researched the question "What can I see on the moon" according to my own ideas, but then I came across Spix & Gasparini's book Moonhopper, which is my most detailed book on the "moon" topic. There, I found the above-mentioned special resolution values for moon structures as well as practical "corrections." Much later in the book (on page 50), the authors discuss the related topic of "optimal magnification." In this chapter, they assume a visual acuity/resolution of the human eye of 1', for which they calculate the beneficial magnifications (while Stoyan assumes 3' for deep sky objects, and most of the information sources on "maximum magnification" assume 2'...). In addition, the authors calculate the beneficial magnifications for moon structures without applying the "practice factor" of 2. I first had to understand all this!

 

What Does the Atik Infinity Camera "See" on the Moon?

This depends, of course, on the telescope used. When I took a photo of the crescent moon with my Explorer 150PDS, the diameter of the moon was about 1080 pixels, which corresponds to 1800" or 3476 km. Thus, 1 pixel corresponds to 1.67" or 3.22 km. This is roughly double the distance that, except for my refractor, my telescopes can resolve. Thus, the Atik Infinity camera does not seem to exploit the resolution of my telescopes (except for the refractor).

A few measurements:

These estimates are plausible. Better accuracy is achieved with large craters. The small craters are used to determine the resolution limit.

 

What Does a Digital Camera See on the Moon?

I took most of my moon photos with a camera held or attached to the eyepiece. Here I discuss two photos taken with the Ricoh GR (16 megapixels, APS-C sensor) on the Skymax-102 and Skymax-127 (for the photos see below).

R0048031 (GR, Skymax-102)

Moon diameter approx. 2000 pixels corresponding to 1800" or 3476 km >> 1 pixel corresponds to 0.9" or 1.74 km
This is more or less the telescope resolution, that is, the camera fits the telescope (the used Skymax-102 has a resolution of 1.15", the 150PDS one of 0.77").

Measurements:

R0048106 (GR, Skymax-127)

Moon diameter approx. 2650 pixels corresponding to 1800" or 3476 km >> 1 pixel corresponds to 0.68" or 1.31 km

This is a little bit better than the telescope resolution, that is, the camera fits approximately the telescope (the Skymax-127 used has a resolution of 0.91", the 150PDS has a resolution of 0.77").

Measurements:

These estimates are plausible. Higher accuracy is achieved with large craters, the small craters are, however, needed to determine the resolution limit.

Detail Photos

After a little more searching and viewing of the original photos I found (preliminarily) out that objects between 5-7 km diameter can be "guessed" and objects of 8 km diameter or more can be recognized (see photos below). This is certainly only true for the better photos like the ones below.

    

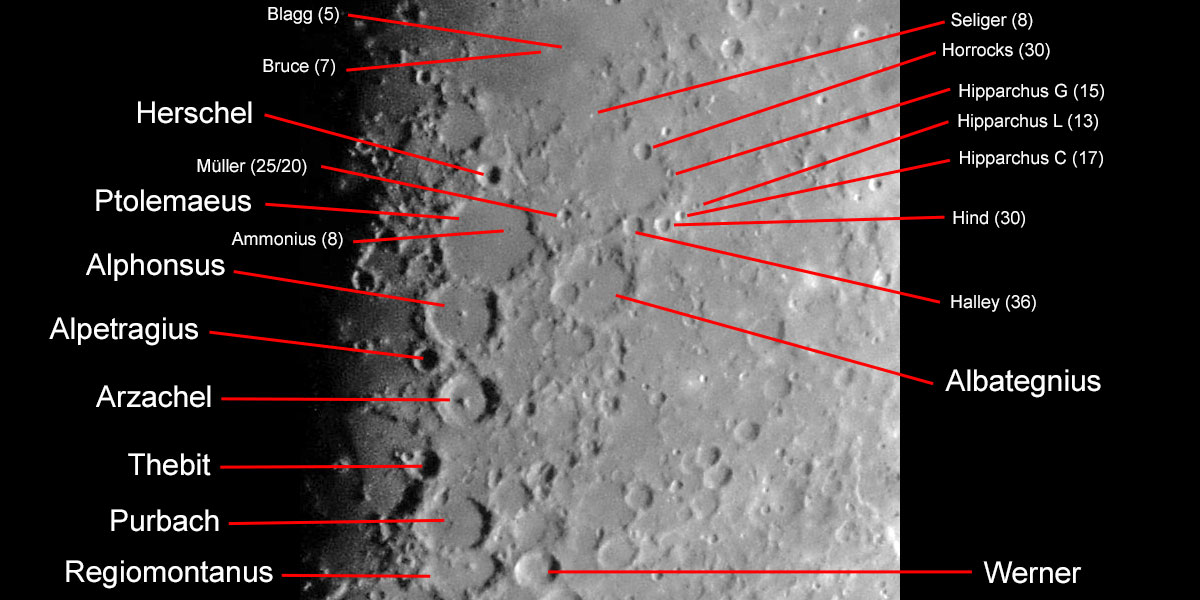100% section

 

Examples of small craters with diameters in km

    

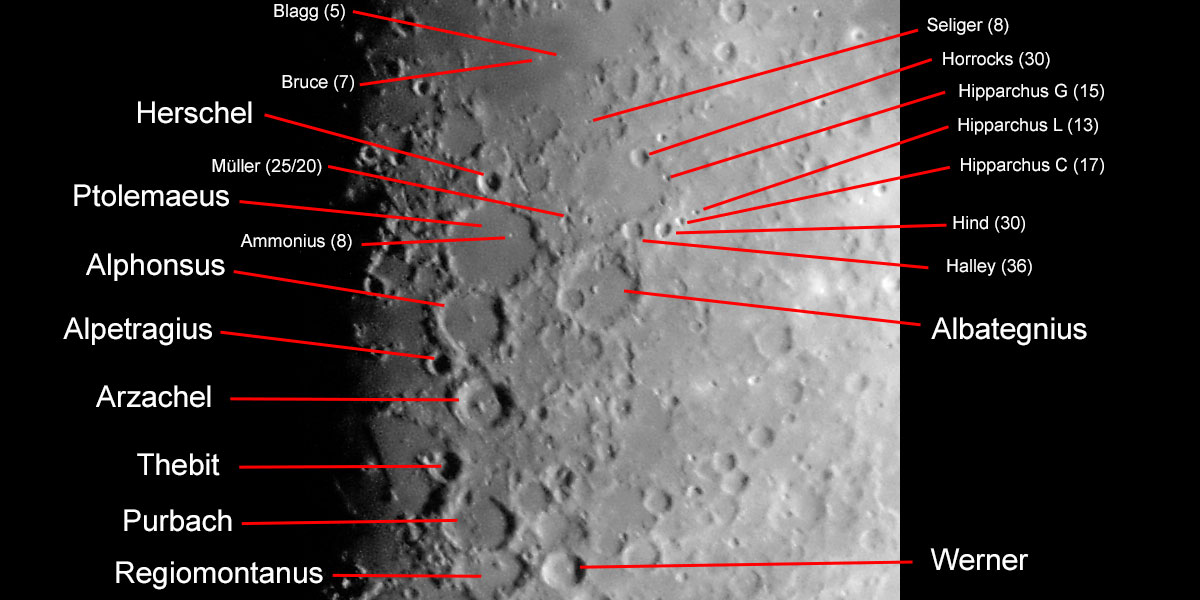100% section

 

Examples of small craters with diameters in km

Photo data: February 23, 2018, Sky-Watcher Skymax-102 telescope, Ricoh GR held to the eyepiece

    

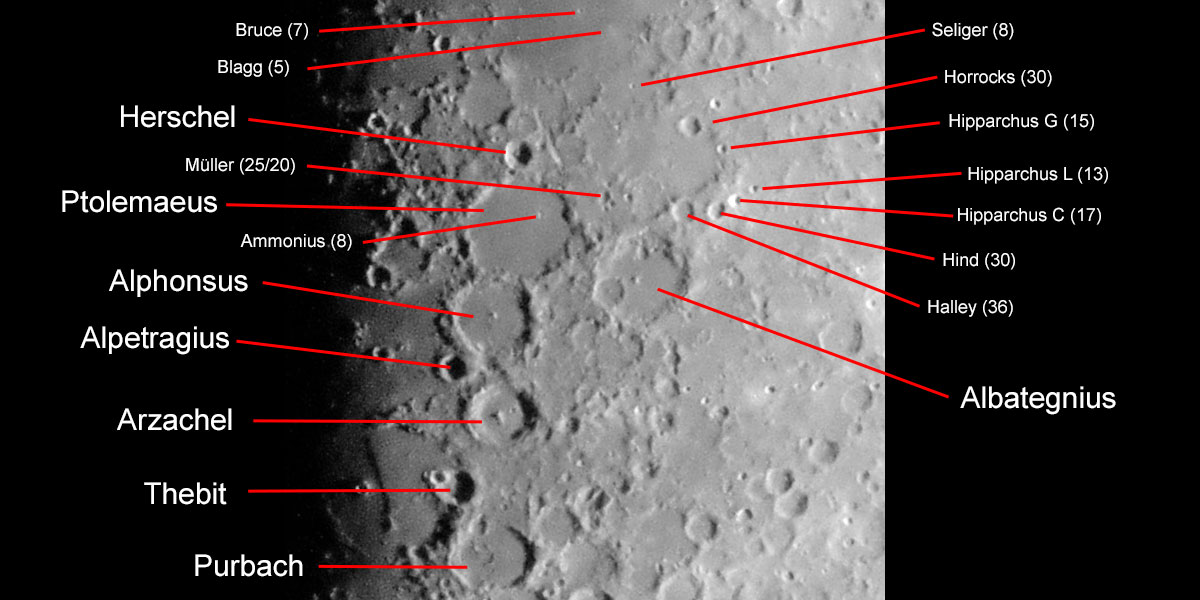100% section

 

Examples of small craters with diameters in km

Photo data: February 23, 2018, Sky-Watcher Skymax-127 telescope, Ricoh GR held to the eyepiece; the shape of crater Müller is reproduced better here than on the photo above

After further searching I found a relatively "easy to find" crater of 5 km diameter (Gassendi Y) on one onf my photos, even though it is only a very faint little dot:

 

What Does a Digital Camera Alone See on the Moon?

I would like to answer this question for my Sony RX10 M3, which has a focal length of 600 mm (equivalent). But with that it just has 20% of the focal length of the Nikon Coolpix P1000, which holds the (equivalent) focal length record as of today (February 2019).

Moon diameter approx. 875 pixels corresponding to 1800" or 3476 km >> 1 pixel corresponds to almost 2.1" or 4.0 km. This is significantly below the resolution of my Omegon PS 72/432 refractor, which has a resolution of 1.61".

On the following photo I believe to recognize the crater Luther, which is, depending upon the source, 9 or 10 km in diameter:

So, I almost achieve what I can achieve with a telescope. Generally, however, it looks as if I can only see a fraction of the craters of this size.

 

References

 

An den Anfang   Homepage  
gerd (at) waloszek (dot) de

About me
made by walodesign on a mac!
06.12.2022